Difference between revisions of "Small Launch Vehicles"
| (154 intermediate revisions by 2 users not shown) | |||
| Line 3: | Line 3: | ||
* '''1SLV - Small Launch Vehicles''' | * '''1SLV - Small Launch Vehicles''' | ||
The Small Launch Vehicles (SLV) market, a level 1 roadmap, is a segment of the global orbital launch services market. Level 2 roadmaps are at the product level (specific launch systems), level 3 roadmaps are at the launcher subsystem level and level 4 roadmaps are at the launcher component level. | |||
==Roadmap Overview== | ==Roadmap Overview== | ||
Small launch vehicles (also called "Small launchers" or "Small-lift launch vehicles") are a category of orbital launchers capable of carrying a payload of maximum 2000kg* to LEO (Low Earth Orbit). Half a century ago, many families of launch vehicles have started as small launchers, as prototypes, and led to today's large landscape of heavy launch vehicles (Ariane V, Falcon 9, Soyuz, Delta IV...). Today, the growing market of small satellites (below 500kg) - including, notably, LEO constellations projects and cubesats - pushes towards dedicated small launchers. This trend started in the | Small launch vehicles (SLVs, also called "Small launchers" or "Small-lift launch vehicles") are a category of orbital launchers capable of carrying a payload of maximum 2000kg* to LEO (Low Earth Orbit). Half a century ago, many families of launch vehicles have started as small launchers, as prototypes, and have led to today's large landscape of heavy launch vehicles (Ariane V, Falcon 9, Soyuz, Delta IV...). Today, the growing market of small satellites (below 500kg) - including, notably, LEO constellations projects and cubesats - pushes towards dedicated small launchers. This trend started in the 1990s and considerably accelerated in the 2010s with the development of a broad ecosystem of SLV startups. In comparison to conventional (heavy) launch vehicles, SLVs have: a higher scheduling flexibility, a higher launch frequency, a much lower total cost but a higher cost per kg of payload. | ||
<gallery class="center"> | |||
File:Categ.jpg|525px|center|Classification of SLVs among launch vehicles on their payload capacity. Source: SpaceCapital. | |||
Ng12timeline.jpg|450px|center|Launch profile of a typical SLV mission with 2 stages and non-reusable rocket (Antares here). Source: Northrop Grumman. | |||
File:Rocketlab2.jpg|415px|center|Launch of the Electron SLV (Rocket Lab). Source: Rocket Lab. | |||
File: Electron illustration.png|270px|center|System decomposition of a SLV (Electron here). Source: Rocket Lab. | |||
</gallery> | |||
'''*''': Diverse definitions exist. Some define small launchers as limited to 1 ton instead of 2 tons, some also define a lower boundary of 500kg under which launchers are called 'micro-launchers'. | |||
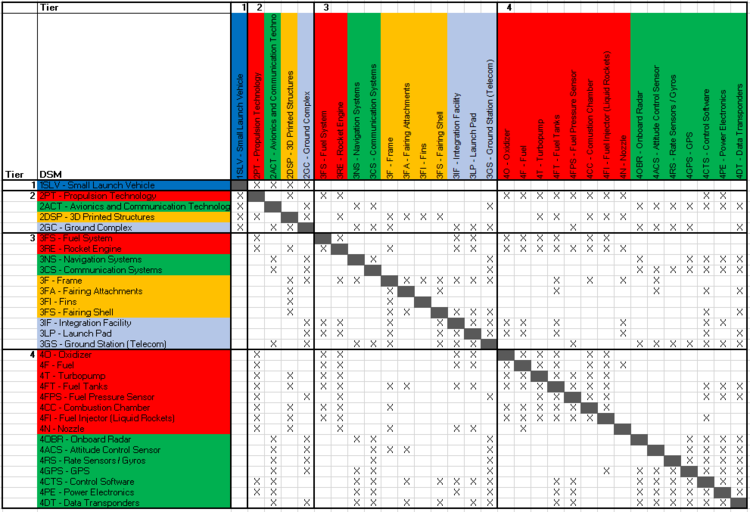
==Design Structure Matrix (DSM) Allocation== | ==Design Structure Matrix (DSM) Allocation== | ||
[[File: | [[File:Hierarchy.jpg|750px|center]] | ||
The | The technology hierarchy demonstrates that such technologies as metal 3-D printing and modulable launchpads are supporting the development of small launchers. Initiatives and development plans for these technologies enhance small launchers by increasing their reusability, launch rate, scheduling flexibility, and reducing their cost per kilogram to launch payloads into orbit. | ||
[[File: | [[File:DSM_v2.PNG|750px|center]] | ||
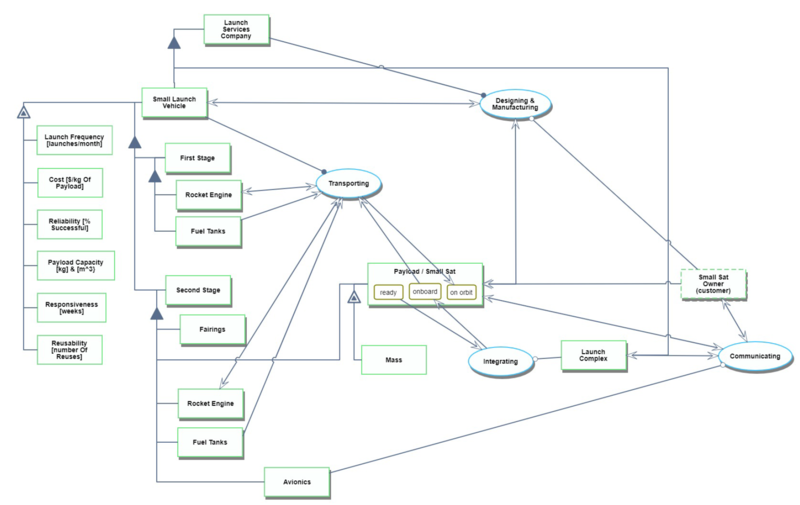
==Roadmap Model using OPM== | ==Roadmap Model using OPM== | ||
We provide an Object-Process-Diagram (OPD) | We provide below an Object-Process-Diagram (OPD) of the 1SLV roadmap. This diagram captures the main object of the roadmap, its decomposition into subsystems (engines, avionics, payload...), its characterization by Figures of Merit (FOMs) as well as the main processes and actors involved. | ||
[[File:Opm.PNG| | [[File:Opm.PNG|800px|center]] | ||
An Object-Process-Language (OPL) description of the | An Object-Process-Language (OPL) description of the technology is auto-generated and given below: | ||
[[File:Opl1.PNG]][[File:Opl2.PNG]] | [[File:Opl1.PNG|400px|center]][[File:Opl2.PNG|400px|center]] | ||
==Figures of Merit== | ==Figures of Merit== | ||
Figures of Merit (FoM) used to evaluate small launchers are very similar to the FoMs | Figures of Merit (FoM) used to evaluate small launchers are very similar to the FoMs used for conventional launch vehicles. However, priorities among these FoMs have changed significantly. Typical FoMs for small launchers are: | ||
{| class="wikitable sortable" style="margin-left: auto; margin-right: auto; border: none;" | |||
|- | |||
! Figure of Merit (FOM) !! Unit !! Description | |||
|- | |||
| Scheduling flexibility || [weeks or months or years] || How quickly the launch vehicle can go from initial talks with customer to launch to LEO. (Sometimes called Responsiveness, it is the duration between the launch window and the notification of its flight parameters - orbit, latitude, date, etc.) | |||
|- | |||
| Launch Frequency || [launches/month] || The number of launches a launch vehicle can perform in a month | |||
|- | |||
| Cost per kg || [$/kg of payload to LEO] || The total cost of the launch divided by the amount of payload to 500km LEO | |||
|- | |||
| Reliability || [% of successful launches] || The percentage of launches out of total launches that are successful as determined by ability to meet end requirements of customers | |||
|- | |||
| Payload mass capacity || [kg of payload to 500km LEO] || The amount of payload in kilograms that can be launched to 500km Low Earth Orbit (LEO) with the launch vehicle. LEO is a large range and so this FOM standardizes the various launch vehicles by specifying 500km LEO | |||
|-w | |||
| Total cost || [$] || The total cost of the launch, which is complementary to the cost per kg as it indicates the price of a ticket for a customer to get a dedicated launch (with dedicated orbit parameters, etc.). | |||
|- | |||
| Reusability || [Number of reuse cycles] || The number of times a launch vehicle can be reused before a new one must be built | |||
|- | |||
|} | |||
In general, small launcher companies aim for very high launch rates (weekly instead of monthly), high scheduling flexibility (months instead of years), and low launch costs thanks to significant economies of scale enabled by mass production. Some also leverage uncommon launch strategies (balloons, planes, catapults), but here we only include into our scope actual orbital launchers with launching pads on earth or on sea. | In general, small launcher companies aim for very high launch rates (weekly instead of monthly), high scheduling flexibility (months instead of years), and low launch costs thanks to significant economies of scale enabled by mass production. Some also leverage uncommon launch strategies (balloons, planes, catapults), but here we only include into our scope actual orbital launchers with launching pads on earth or on sea. | ||
The following plots have been built using a database our team built and plan upgrade regularly throughout time. Main sources of information are SpaceFund.com, Newspace.im and small launcher companies' websites and user guides. | |||
Evolution of the *cost per kg* of Small Launch Vehicles over time, for both existing and planned concepts: | |||
[[File:Last edit.png|700px|center]] | |||
[[File:Rate.PNG|700px|center]] | |||
The physics of launchers is governed by the Rocket Equation: | |||
$$\Delta V = v_e \log(\frac{m_0}{m_f})$$ | $$\Delta V = v_e \log(\frac{m_0}{m_f})$$ | ||
$\Delta V$ is the change of | <math> \Delta V </math> is the change of velocity available to the missile | ||
<math> m_0 </math> is the initial wet mass | |||
<math> m_f </math> is the (final) dry mass | |||
<math> v_e </math> is the effective exhaust velocity | |||
==Alignment of Strategic Drivers== | |||
We see the competitiveness of the SLV market to be soon dictated by launchers' scheduling flexibility and launch frequency, but with cost per kilogram still as a significant figure of merit. With this in mind, our SLV company aims to allow, first, the highest scheduling flexibility (shortest time between customer contact to launch) and, second, as affordable as possible in these conditions. | |||
{| class="wikitable" | |||
|- | |||
! Number !! Strategic Objective !! Alignment and Target(s) | |||
|- | |||
| 1 || A scheduling flexibility that allows a customer to launch in the shortest time frame after the 1st contact|| The current average is ~2 years. A competitive average would be a required time of ~1.5 years. A dominant average would be 6 months. We aim to first reach the scheduling flexibility of 6 months, eventually aiming to achieve a required planning time of only 4 weeks. | |||
|- | |||
|- | |||
| 2 || A launch frequency that allows us to launch as many payloads from as many customers as possible || The current average is ~2-3 launches per year . A competitive average would be ~6 launches per year. A dominant average would be 10 launches per year. We aim to reach the launch frequency of 10 launches per year. | |||
|- | |||
| 3 || A total cost that is affordable by even small, academic/organizational endeavors with modest budgets || We aim to be able to perform any launch with a cost per kilogram under $16,000 and a total cost under $11,500,000 (400kg payload at $16,000/kg and $5,000,000 of fixed costs | |||
|} | |||
FOMs such as reliability and mass to LEO are not included for a reasons. Reliability is of course a significant consideration, but not necessarily a strategic driver that is pursued per se. Of course, with any operations such as rocket launches that have considerable overhead costs and risks of catastrophic failure (entire system loss) it is important to reduce costs risks however possible. However, maximizing reliability and mass to LEO always helps but is not a primary driver of being successful in extracting value from the small launch market. | |||
==Positioning of Company versus Competition== | |||
[[File:LaunchVehicleProviderMarket.JPG|950px|center]] | |||
The first graph depicts the positioning of the small launcher market with respect to the medium, heavy, and super-heavy launch markets. Larger launchers, such as SpaceX's Falcon 9 rocket, are able to provider cheaper costs per kilogram with high reliability which explains their positioning as cheaper producers and improved value. Small launchers on the other hand cost more per kilogram than the other launchers but the launch frequency as well as the responsiveness are improved. | |||
The micro- and nano- launch markets are much smaller and unproven so therefore not shown. However, the value of those two markets would be more niche in providing smaller, more specified payloads and thus more expensive with respect to $/kg albeit it at the tradeoff of likely higher launch frequencies and lower overall costs. | |||
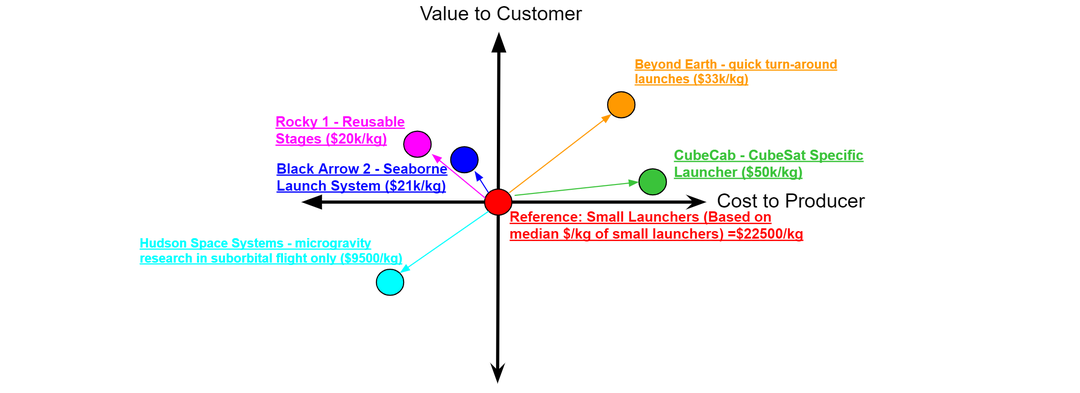
[[File:SLVMarketingPosition.png|1080px|center]] | |||
The above graph depicts the positioning of a standard small launch company (based upon the average small launcher cost per kilogram (average frequency of launch is difficult to assume due to low available data but would be a better reference and indicator of value) based on the suggested FOM introduced by small launchers. "Value" in this sense is a combination of various FOMs such as launch frequency and launch costs ($/kg). It is weighted heavily toward launch frequency as this is the primary FOM for small launchers, although launch cost is still significant. As seen, various small launch vehicle providers are attempting to bring value through respectively unique means. Some are attempting to introduce reusable stages similar to SpaceX's Falcon 9 to small launchers, others are attempting to narrow in on the niche of cubesats, some are attempting to focus on seaborne launch which reduces the costs of aborting launch and clearing an area/waiting for weather, and so on. Although some competitors are increasing costs in order to accommodate more niche markets while others are pursuing more advanced, riskier technologies to reduce overall costs, all are attempting to differentiate their service from other competitors in order to extract value from their own niche and remain profitable in their own competitive space. | |||
Small Launcher Cost Per Kilogram and Launch Frequency Data Table | |||
{| class="wikitable sortable" style="margin-left: auto; margin-right: auto; border: none;" | |||
|- | |||
! Launcher !! Launches Per Year !! Cost Per Kilogram ($/kg) | |||
|- | |||
| Vector-R || 100 || 25000 | |||
|- | |||
| Electron || 52 || 39300 | |||
|- | |||
| Bagaveev || 50 || 25000 | |||
|- | |||
| Alpha || 24 || 15000 | |||
|- | |||
| StarLord || 12 || 20000 | |||
|- | |||
| Miura 5 (Arion 2) || 10 || 32500 | |||
|- | |||
| Pythom || 2 || 12000 | |||
|- | |||
| OS-M || 5 || 16000 | |||
|- | |||
| Pegasus || 6 || 180586 | |||
|- | |||
| Kuaizhou-1A || 5 || 20000 | |||
|- | |||
| JAXA SS-520 || 0.5 || 31429 | |||
|} | |||
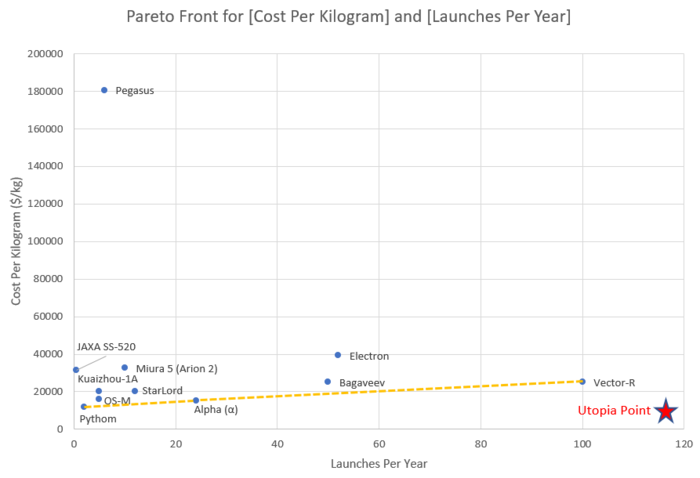
[[File:SmallLauncherPositioning4.PNG|700px|center]] | |||
The pareto front above is not based on time but rather cost per kilogram and launches per year (innate with as launch frequency/scheduling flexibility). As seen, the Pythom, Alpha, and Vector-R launchers are pareto optimal designs whereas the other launchers are dominated alternatives. Of note, however, is that many, many launchers (150+, 50 of which are pictured below) did not have data publicly available on both Figures of Merit and therefore were omitted from the data set. Furthermore, some launchers such as Pythom, are still in the developmental stages are projecting these launch costs and frequencies despite having never operationally proven these figures. Other launchers, some as the SS-520, Pegasus, and Kuaizhou-1A have been operationally proven with several successful launches. Currently, small launch companies are being reached out to manually in order to inquire about projections and more data is being continually added to the set as they respond. The current pareto optimal alternatives are expected to shift as more are added and the model can be improved by differentiating between pareto optimal launchers that are operational and those that are not yet launched. Finally, at $22,500, the reference company above would be pareto dominated by all other small launchers currently on the market. This means that the reference company should focus on both decreasing launch costs and increasing launch frequency. | |||
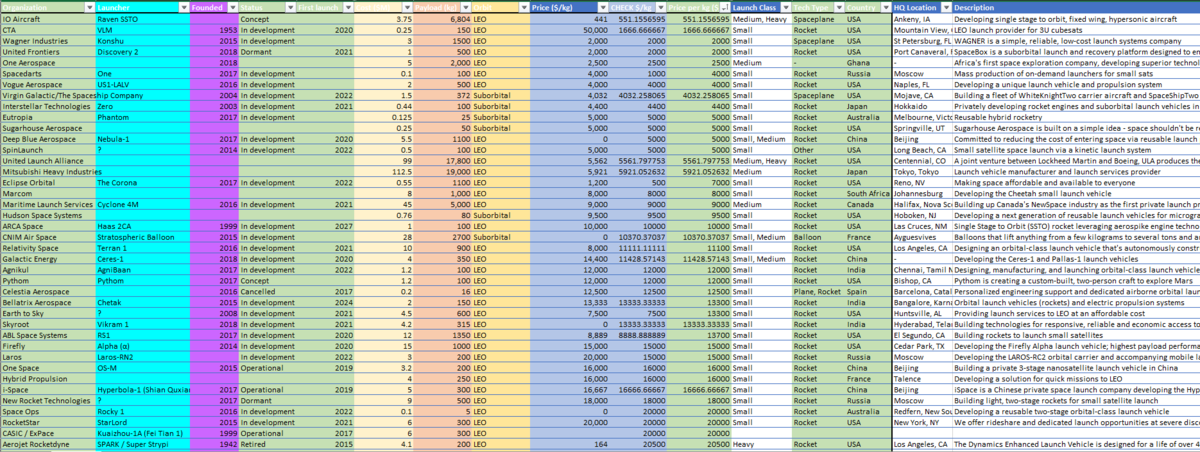
Our database of competing SLV providers: | |||
[[File:DataTableScreenshot.PNG|1200px|center]] | |||
==Technical Model: Morphological Matrix and Tradespace== | |||
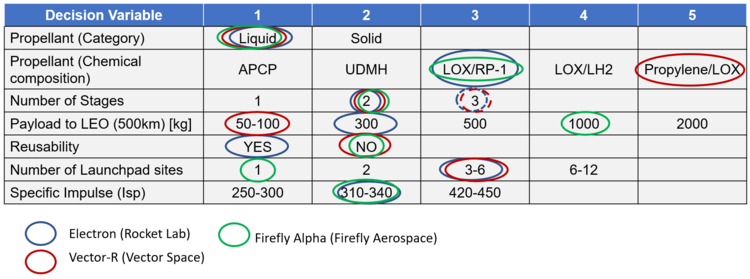
Morphological Matrix: | |||
[[File:MorphoM1.PNG|750px|center]] | |||
The *Scheduling Flexibility* of SLVs (Small Launch Vehicles) is by far the main differentiator of this market segment. Building such a model for responsiveness (which is based on organizational principles rather than physical principles) is complex and very little data is available at this granularity. For these reasons we decomposed this figure of merits into a sum of components on which we have actual data, or can do an educated guess with limited uncertainty in terms of factors at play and relative magnitudes. We used the SLV databases already mentioned in previous sections, manufacturers' websites, and for data only available for heavy launch vehicles, we scaled them. | |||
For simplicity, we decide to use ***lead time*** as a proxy for Scheduling Flexibility: it is defined as the average duration between the order from the customer and the actual launch provided by the SLV services company. It assumes that a contract already exists between the two sides, but the chronometer starts when the actual orbit parameters, launch date and other specifications are communicated. Delays are very frequent, so we decompose responsiveness in the following way: | |||
Lead time (days): | |||
$$R = \sum D_{\text{Launch Prep. Phases}} + \sum P_{\text{Delay Likelihood}} \cdot D_{\text{Delay}}$$ | |||
$$R = D_{1} + D_{2} + D_{3} + D_{4} + D_{5} + D_{6} + P_L D_{L-Delay} + P_S D_{S-Delay}$$ | |||
With (in Days): | |||
S/C mating onto adapter: $$D_{1} = 3 \frac{m}{2000} $$ | |||
S/C mating into launcher: $$D_{2} = 5 \frac{m}{2000}$$ | |||
Fairing installation $$D_{3} = 1 $$ | |||
Launchpad preparation: $$D_{4} = 7 + 3 N + 2 S$$ | |||
S/C-Launcher Mission definition (trajectory, perf...): $$D_{5} = 15 - \frac{N}{2} - \frac{3}{2} S$$ | |||
Fuel system of main stage: | |||
$D_{6} = 2$ for SRMs (solid rocket motors) ; $D_{6} = 6$ for liquid rocket engines | |||
Delays: | |||
- Launcher | |||
Likelihood: $$P_L= 0.3*\frac{m}{2000} + P_1 + P_2 $$ | |||
with <math> P_1 = 0.05 </math> if reused launcher (0 otherwise) and $P_2 = 0.1$ for liquid rocket engines (0 otherwise). | |||
Delay: $$D_{L-Delay} = 10$$ | |||
- Satellite/Spacecraft S/C | |||
Likelihood: $$P_S= 0.05*\frac{m}{2000} $$ | |||
Delay: $$D_{L-Delay} = 20$$ | |||
Parameters: | |||
m payload mass capacity (mass of the spacecraft, S/C) 0<m<=2000kg | |||
N number of launchpad sites available (hypothesis: optimized spreading among the conventional inclination values used for LEO missions). 0<N<=8 | |||
S number of rocket stages 0<S<=3 | |||
“𝛿0” Disposable vs reused launcher main stage | |||
“𝛿1” Propellant type for main stage (solid vs liquid) | |||
Comments: | |||
Phases that can be conducted in parallel of other “constraining” tasks (i.e. on the critical path of the supply chain) have been included in phases done in series. | |||
N is a variable that describes the ability of the company to launch from different sites at different inclinations, whether it is a fixed launchpad or a movable one (the more launchpad sites available, the easier the mission definition phase, the shorter the wait for an available site, but the less ready to go) | |||
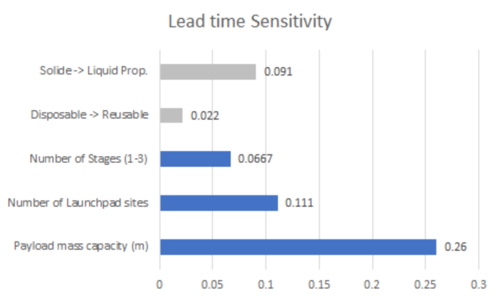
[[File:TornadL.png|500px|center]] | |||
As expected, in order to improve the responsiveness (reduce the lead time) of a SLV, it should have: | |||
- a lower mass capacity | |||
- a lower number of launchpads (but it will restrain of course the type of missions feasible) | |||
- a lower number of stages (2, or sometimes 1, instead of 3 for heavy launch vehicles, but it will restrain of course the type of orbit insertions feasible) | |||
- Solid propulsion instead of liquid propulsion (which has other inconvenients, such as no restartability) | |||
- Disposable main stage (used launchers have generally more unexpected failure, but will save a lot of money on the long term). | |||
Another important FoM for any rocket is its ***Delta V***, which will enable it to reach specific orbits. In the case of SLVs, some rockets will be able to reach 300-400km LEO orbits, while others reach up to more than 1000km LEO orbits. SLVs are usually unable to reach MEO or GEO orbits (Medium Earth Orbit or Geostationary orbit) with they typical payloads. | |||
Rocket equation (neglecting drag and gravity losses): | |||
$$\Delta V = \text{Isp} \cdot g_0 \log (R)$$ | |||
Parameters: | |||
- Isp the specific impulse of the SLV, which is a measure of how effectively the SLV uses its propellant | |||
- R = M0/Mf is the mass ratio of our rocket, M0 being the wet mass (mass including propellant) and Mf the dry mass (final mass, with all propellant consumed) | |||
$$ \frac{\partial \Delta V}{\partial \text{Isp}} = g_0 \log (R)$$ | |||
$$ \frac{\partial \Delta V}{\partial R} = \frac{\text{Isp} \cdot g_0 }{R}$$ | |||
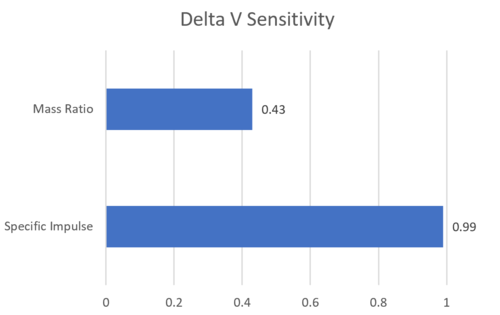
$$ \frac{Isp_0}{\Delta V_0} g_0 \log (R_0) = 0.99$$ | |||
$$ \frac{R_0}{\Delta V_0} \frac{\text{Isp_0} \cdot g_0 }{R_0} = 0.43 $$ | |||
[[File:Deltav.PNG|500px|center]] | |||
==Financial Model== | |||
This financial model is performed from the perspective of our launch vehicle services company (model details and hypotheses are stated below): | |||
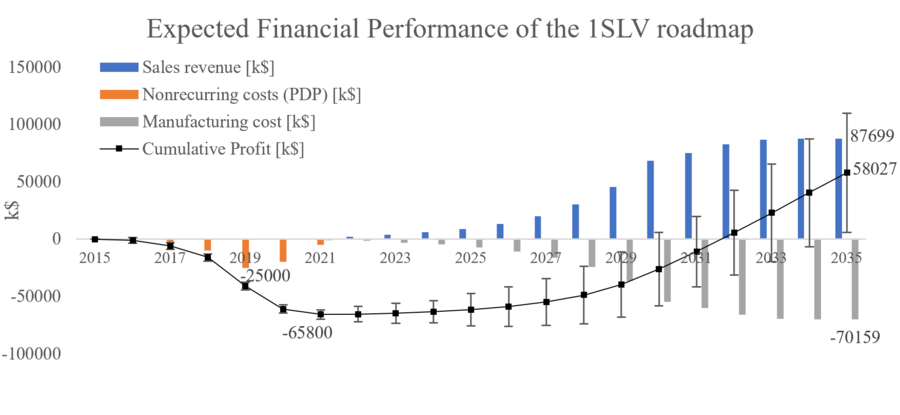
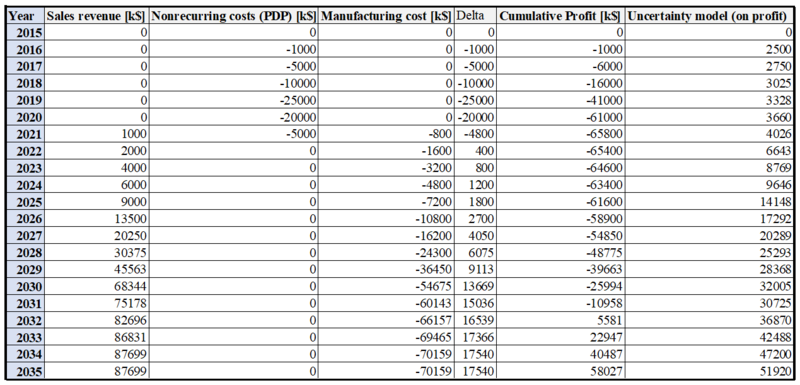
[[File:Fin graph2.PNG|900px|center]] | |||
The breakdown of the expected financial performance brought by the 1SLV roadmap is as follows: | |||
* Annual profit and cumulated profit are derived from sales revenue and costs: | |||
** Revenues | |||
*** Revenue sources are contracts with satellite operators to launch their payloads to orbit. Thanks to the responsiveness of our launch vehicle, we assume in our model that the whole payment for a launch is processed within a timeframe of one year (from initial deposits to final payment close to launch date). | |||
*** Revenue directly depend on the number of launches performed every year. It is modeled as a slow ramp-up phase (prototype, with lower revenue to account for the high risk of failure), then a phase of acceleration, then convergence to the size of the market segment addressed by our company. Prices have been taken from the analyses conducted above, from our launchers database, and when necessary from the Rocket Lab electron rocket. | |||
** Costs | |||
*** Manufacturing cost (variable): modeled in steady range as 80% of the price of the launcher (for the customer - data from electron), with an initial phase of high manufacturing cost to account for growing economies of scale. They start in 2021 with the manufacturing of the first launchers. | |||
*** Nonrecurring costs (fixed): investments to develop this new small launch vehicle leveraging the technologies explained above. We assume that the product development process (PDP) was started earlier in 2016, and investments were done over a 6-year period. 2021 is a year of transition where there are still final PDP investments along with variable costs for the manufacturing of the first launchers. | |||
This financial model is of course a projection, that should be accompanied by an uncertainty level (see error bars on the graph above). We built a simple error model which accounts for uncertainty in development costs, sales and manufacturing costs. The uncertainty on sales is the main driver of the overall uncertainty level, followed by uncertainty in PDP-related investments, and finally manufacturing costs. | |||
Main insights from the financial analysis: | |||
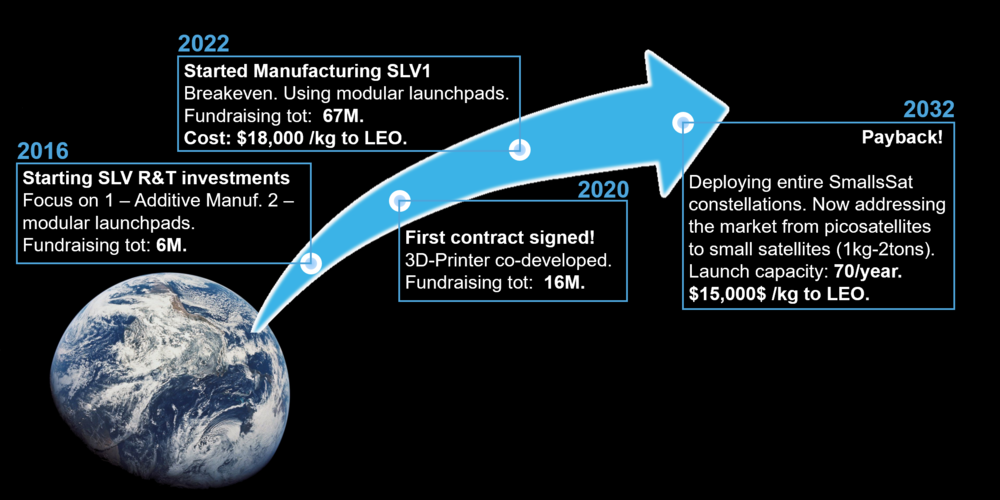
* The average financial scenario is profitable in 2032, which is long but reasonable for this very specific market of launch vehicles with huge initial investments. It is actually close in timeframe to what was announced by Vector Lab. In the worst case scenario considered, profits only exceed upfront investments in the year 2035 (lower boundary of the last cumulative profit estimate). | |||
* Breakeven happens in 2022, second year after starting manufacturing the rockets | |||
Hence the financial projections (also shown on the graph above): | |||
[[File:Finance2.PNG|800px|center]] | |||
==List of R&T Projects and Prototypes== | |||
'''Summary''' | |||
The choice of R&D projects to undertake is driven by two different sets of priorities. First, our strategic drivers of increased scheduling flexibility and decreased total cost. Second, those that improve secondary FoMs: launch frequency (launches/month), reliability (% successful launches, payload mass capacity (kg to 500km LEO), and reusability (# of reuse cycles). | |||
<gallery class="center"> | |||
AdditiveManufacturing.jpg|Additive Manufacturing | |||
ModularLaunchPadPlease.jpg|Modular Launchpad | |||
SLVCaseMaterials.png|Case Materials | |||
ScramjetExample.jpg|Rocket-Based Combined-Cycle (Scramjet) | |||
PredictiveFailure.png|Models and Approaches for Remaining Useful Life Prognostics | |||
</gallery> | |||
'''R&D Projects''' | |||
{| class="wikitable sortable" style="margin-left: auto; margin-right: auto; border: none;" | |||
|- | |||
! R&D Project !! Technology Area !! Description !! Pros !! Cons !! Invest? Y/N | |||
|- | |||
| Additive Manufacturing || Materials || 3D-Printing of rocket components out of metal alloys || | |||
* Cheaper manufacturing of complex pieces | |||
* New component designs become manufacturable | |||
* Shorter manufacturing process (less steps and time) | |||
|| | |||
* High upfront investment in expensive printers | |||
* Specific expertise required | |||
* Lack of experience with this technology can lead to unknown design flaws | |||
|| | |||
Y | |||
|- | |||
| Modular Launchpad || Vehicle Integration || Allows easier integration of launch vehicles into launchpads || | |||
* Easier integration of launch vehicle to launch pad | |||
* Lower costs | |||
* Increased scheduling flexibility | |||
* Increased launch frequency | |||
|| | |||
* Potentially design limiting for customers | |||
* The improvement in each of these categories is small | |||
|| | |||
Y | |||
|- | |||
| Case Materials || Rigging and Load Integration || Improvement of materials and composites and attachments used in the vessel of the rocket to allow easier integration of payloads and lower mass vehicles as well as more quick, reliable, and/or resilient fabrication || | |||
* Reduced Weight | |||
* Reduced Cost | |||
* Reduced Schedule | |||
* Easier integration of payloads | |||
|| | |||
* The improvement in each of these categories is small | |||
|| | |||
Y(/N) | |||
|- | |||
| Rocket-Based Combined-Cycle || Propulsion System || These projects integrate ramjet and/or scramjet technology with rocket launches in order to improve acceleration to orbit. || | |||
* Reduced Cost | |||
* Reduced Schedule | |||
* Increased Scheduling Flexibility | |||
|| | |||
* Much higher complexity (decreased reliability) | |||
* Low Current TRL (3) | |||
* Decreased payload mass to orbit | |||
* Low reusability in rocket, high reusability in plane (still cheaper overall) | |||
|| | |||
N | |||
|- | |||
| Models and Approaches for Remaining Useful Life Prognostics || Prognostic || Allows component level measurement and estimation/prediction of time to failure so that forecasting and mitigation of failures may occur within an acceptable confidence level (90-99%) || | |||
* Improved reusability | |||
* Increased launch frequency (less time manually inspecting components) | |||
* Increased scheduling flexibility (less time manually inspecting components) | |||
* Moderate TRL for basic models with 90% confidence level (6) | |||
|| | |||
* The improvement in each of these categories is small | |||
* Low TRL for Machine Learning prognostics with 99% confidence level (3) | |||
* Difficult to continually update as new components come into service or paradigms change (e.g. in the event air launches become commonplace) | |||
|| | |||
N | |||
|- | |||
| Air Launch and Drop System || Plane Launch || Uses fighter or airliner planes to reach a higher altitude before launching the attached payload || | |||
* Reduced Cost | |||
* Reduced Schedule | |||
* Increased Scheduling Flexibility | |||
|| | |||
* Low TRL (5) | |||
* Decreased payload mass to orbit (~<100kgs or only nano-sats) | |||
* Low reusability in rocket, high reusability in plane (still cheaper overall) | |||
* Slightly higher complexity (decreased reliability) | |||
|| | |||
N | |||
|- | |||
|} | |||
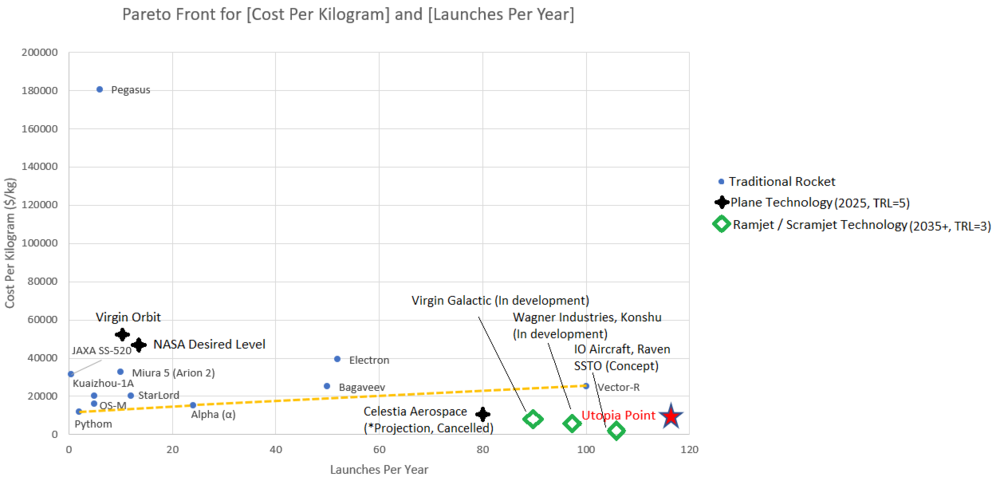
A comparison of the R&D projects that are projected to alter the FOMs significantly, the scramjet and air launch projects, is below. Compared to traditional rockets, they appear to come vastly closer to the utopia point. However, there are no legitimate, successful tests of either of these. Although the prospects are promising, the TRL is simply too low and the cost of investment to increase this to operational capabilities is too high. However, as the TRL increases through research currently being performed by other agencies, primarily the Department of Defense, we recognize these technologies as potentially threatening to the small launch vehicle market space we currently operate in. | |||
[[File:PleaseWork.png| 1000px |center]] | |||
Other R&D projects - prognostics model, case materials, and modular launchpad - have higher TRLs but have less significant impacts on the key FOMs, most importantly the scheduling flexibility and total cost. The prognostic model is being developed by other companies that also incorporate reusable boosters as this technology would be particularly beneficial to assess the reused boosters. Since there are no plans to explore the reusable booster technology space, we do not see this an optimal choice to invest in currently. | |||
Investing in case materials and a modular launch pad are the most lucrative as these contribute to the FOM most relevant to our business model of appealing to consumers that would prefer to be able to launch on demand rather than wait months/years for cheaper ridesharing options. A modular launchpad and revised case materials allow clients to more easily integrate payloads into our launch vehicles, thereby resulting in increased scheduling flexibility (shorter time between initial talks to launch). As our primary FOM and a central aspect to our business model, the benefits yielded from both of these R&D projects that are also relatively inexpensive to design and develop, warrant the further examination below. | |||
[[File:CanIUploadThisPlease.png| 800px |center]] | |||
[[File:R&DParetoFrontier.png| 600px |center]] | |||
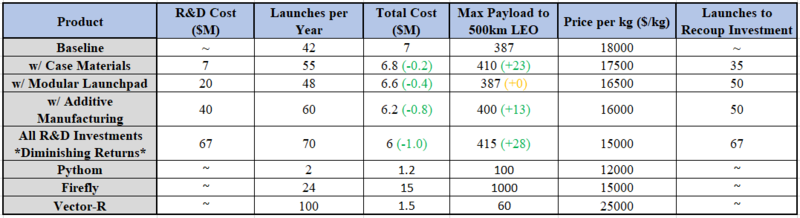
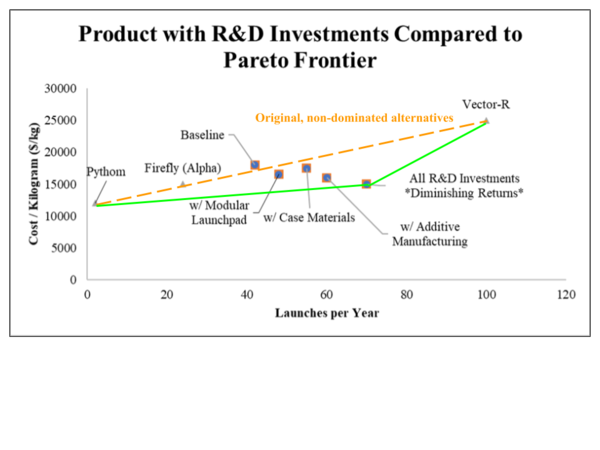
The values are gathered from current cost estimations for related technologies combined into a nominal projected value. For example, the cost estimation for a modular launchpad was based on the current TRL of modular launchpads based upon NASA publications, the current cost of a launchpad, and the current cost of similar R&D projects for materials and structures research. It should be noted that '''we assume diminishing returns when all three R&D projects are incorporated simultaneously'''. This is due to some overlap such in the improvements associated with each. For example, case materials may overlap with the materials science involved in additive manufacturing. Our company's technology baseline is a rounded down average of the three pareto dominant alternatives analyzed above: Pythom, Firefly (Alpha), and Vector-R. This makes us near competitive, but just under pareto dominant without any R&D investments. In order to reach pareto dominance, and by extension a competitive market position, we chose to invest in the aforementioned R&D projects with higher TRLs that can become operational in a shorter amount of time. This, in turn, allows us to recoup our investment more quickly. The cost estimations for these projects are based on data available from other sectors attempting to incorporate such projects into their own products (e.g. additive manufacturing in robotics, planes, and construction yielded baseline data for us to estimate the cost and value added for the small launch market). Our pre-research hypothesis that the value-added from these projects is low seems to be correct as the pareto frontier shifts only slightly. However, this shift is enough to exclude Firefly (Alpha) from being a pareto dominant competitor and makes our costs competitive with the others launch providers. It can also be observed that our R&D costs associated with becoming a pareto dominant competitor can be recouped in just one year of launched, assuming we are able to complete all 70 launches expected. | |||
==Key Publications and Patents== | |||
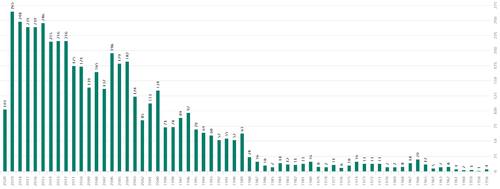
Number of publications per year about small launch vehicles (and equivalent denominations): | |||
[[File:SLVPublicationsPerYear.png|500px|center]] | |||
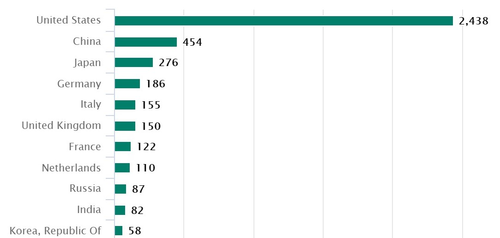
Most families of launch vehicles started as small launcher prototypes, as early as the 50s/60s, hence the few papers from that period. However, small launchers really became an attractive design in the 90s with the start of the miniaturization of satellites. There is a growing number of publications every year on the topic. If we conduct an analysis of author affiliations, we note that JPL (CA) is by far the most active on this topic, followed by the China Academy of Launch Vehicle Technology, and NASA. The countries of origin are the following: | |||
[[File:SLVPublicationLocation.png|500px|center]] | |||
We decided to focus on two papers about small launch vehicle technologies, highly cited and giving a good overview of current research areas. The first focuses on the use of small launchers for cubesat constellations’ deployment, the second one focuses on vibration isolation to protect payloads during launch from SLVs. | |||
'''“Utilizing Small Launch Vehicles for Multiple Small Payload Missions” (2017)''' | |||
Sending several cubesat payloads with different orbital parameters in a single launch is a challenge today because these often don’t have on-board propulsion systems and because small rockets don’t have 3rd/4th stages to precisely insert satellites one at a time. This paper presents a concept for a low-cost/low-mass “propulsive rideshare adapter” called SL-OMV that is able to remain on orbit for longer durations to distribute cubesats up to 6U to their respective LEO orbits. Both authors are employees of Moog, Inc (Aerospace and Defense company). Their solution may be particularly helpful for the deployment of cubesat constellations. | |||
[[File:SLOMVPic.PNG|500px|center]] | |||
SL-OMV from below (left) and integrated under the fairings (right). SL-OMV Capacities. | |||
'''“Multi-axis whole-spacecraft vibration isolation for small launch vehicles” (2001)''' | |||
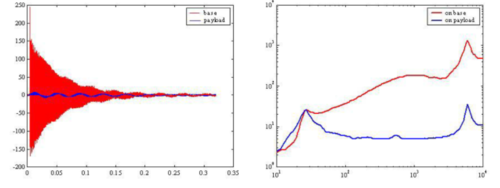
Vibration isolation systems are critical for launch vehicles to protect their sensitive payloads from vibrations resulting from the launch. Because of the mass and volume constraints on small launch vehicles, conventional isolation systems cannot be used. This is clearly a threat to the attraction of small launcher vehicles. This paper presents two passive vibration isolation systems to reduce dynamic launch loads. The first one protects against axial loads and has been used in three launches to date. The second one is a multi-axis device that performs the same functions and has been tested twice for space missions. They are both relatively simple to manufacture, passive, small, lightweight and reliable. | |||
[[File:AttenuationPic.PNG|500px|center]] | |||
Additional attenuation provided by the second isolation device (accelerations/frequencies). | |||
'''Patent analysis''' | |||
We have found patents from small launch vehicle startups, aerospace companies and agencies about propulsion technologies, avionics, control software, launch pads and manufacturing methods. Most patents were filed during the past two decades. The first patent shows a way for SLV manufacturers to cut costs associated with high-quality avionics systems onboard launchers. The second one provides a mechanism to save mass and volume in the satellite deployment system for SLVs. | |||
'''“Affordable vehicle avionics system”, 2020, NASA''' | |||
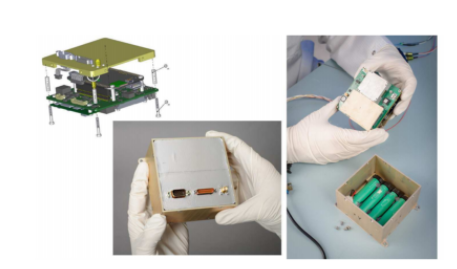
Developed at NASA Ames research center, the AVA (Affordable Vehicle Avionics) is a low-cost, self contained GNC (Guidance, Navigation and Control) system made for nano- and micro-launchers. This system is qualified to survive the launch environment and leverages already existing commercial sensors and processors, to make it cost-effective (10% of the current cost of such systems). Its size is 100 mm x 120 mm x 69 mm with a mass of 0.84kg, it includes a tactical-grade MEMS inertial measurement unit that leverages a GPS receiver, inertial sensors and a magnetometer to reach precision levels suited for space navigation. | |||
[[File:CADPic.PNG|500px|center]] | |||
CAD model and pictures of the AVA (NASA website) | |||
'''“Triggered satellite deployment mechanism”, 2016, Vector Launch Inc.''' | |||
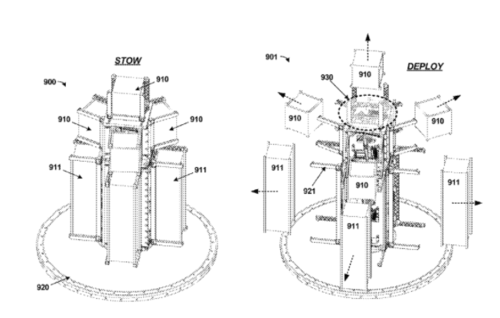
This patent presents an improved design for payload deployment systems. Weight and size are even more critical for small launch vehicles than for traditional launch vehicles, so this patent minimizes the size of the deployment mechanism. More precisely, it avoids having to enclose the satellite in a box structure. It instead uses pairs of “opposing hooks that slide over a set of small chassi rails” that stick out and are compliant with cubesat standards. | |||
[[File:SatDeploymentMechanism.PNG|500px|center]] | |||
Satellite deployment mechanism | |||
==Technology Strategy Statement== | |||
[[File:SwooshX2a.PNG|1000px|center]] | |||
Latest revision as of 20:33, 2 December 2020
Technology Roadmap Sections and Deliverables
- 1SLV - Small Launch Vehicles
The Small Launch Vehicles (SLV) market, a level 1 roadmap, is a segment of the global orbital launch services market. Level 2 roadmaps are at the product level (specific launch systems), level 3 roadmaps are at the launcher subsystem level and level 4 roadmaps are at the launcher component level.
Roadmap Overview
Small launch vehicles (SLVs, also called "Small launchers" or "Small-lift launch vehicles") are a category of orbital launchers capable of carrying a payload of maximum 2000kg* to LEO (Low Earth Orbit). Half a century ago, many families of launch vehicles have started as small launchers, as prototypes, and have led to today's large landscape of heavy launch vehicles (Ariane V, Falcon 9, Soyuz, Delta IV...). Today, the growing market of small satellites (below 500kg) - including, notably, LEO constellations projects and cubesats - pushes towards dedicated small launchers. This trend started in the 1990s and considerably accelerated in the 2010s with the development of a broad ecosystem of SLV startups. In comparison to conventional (heavy) launch vehicles, SLVs have: a higher scheduling flexibility, a higher launch frequency, a much lower total cost but a higher cost per kg of payload.
*: Diverse definitions exist. Some define small launchers as limited to 1 ton instead of 2 tons, some also define a lower boundary of 500kg under which launchers are called 'micro-launchers'.
Design Structure Matrix (DSM) Allocation
The technology hierarchy demonstrates that such technologies as metal 3-D printing and modulable launchpads are supporting the development of small launchers. Initiatives and development plans for these technologies enhance small launchers by increasing their reusability, launch rate, scheduling flexibility, and reducing their cost per kilogram to launch payloads into orbit.
Roadmap Model using OPM
We provide below an Object-Process-Diagram (OPD) of the 1SLV roadmap. This diagram captures the main object of the roadmap, its decomposition into subsystems (engines, avionics, payload...), its characterization by Figures of Merit (FOMs) as well as the main processes and actors involved.
An Object-Process-Language (OPL) description of the technology is auto-generated and given below:
Figures of Merit
Figures of Merit (FoM) used to evaluate small launchers are very similar to the FoMs used for conventional launch vehicles. However, priorities among these FoMs have changed significantly. Typical FoMs for small launchers are:
| Figure of Merit (FOM) | Unit | Description |
|---|---|---|
| Scheduling flexibility | [weeks or months or years] | How quickly the launch vehicle can go from initial talks with customer to launch to LEO. (Sometimes called Responsiveness, it is the duration between the launch window and the notification of its flight parameters - orbit, latitude, date, etc.) |
| Launch Frequency | [launches/month] | The number of launches a launch vehicle can perform in a month |
| Cost per kg | [$/kg of payload to LEO] | The total cost of the launch divided by the amount of payload to 500km LEO |
| Reliability | [% of successful launches] | The percentage of launches out of total launches that are successful as determined by ability to meet end requirements of customers |
| Payload mass capacity | [kg of payload to 500km LEO] | The amount of payload in kilograms that can be launched to 500km Low Earth Orbit (LEO) with the launch vehicle. LEO is a large range and so this FOM standardizes the various launch vehicles by specifying 500km LEO |
| Total cost | [$] | The total cost of the launch, which is complementary to the cost per kg as it indicates the price of a ticket for a customer to get a dedicated launch (with dedicated orbit parameters, etc.). |
| Reusability | [Number of reuse cycles] | The number of times a launch vehicle can be reused before a new one must be built |
In general, small launcher companies aim for very high launch rates (weekly instead of monthly), high scheduling flexibility (months instead of years), and low launch costs thanks to significant economies of scale enabled by mass production. Some also leverage uncommon launch strategies (balloons, planes, catapults), but here we only include into our scope actual orbital launchers with launching pads on earth or on sea.
The following plots have been built using a database our team built and plan upgrade regularly throughout time. Main sources of information are SpaceFund.com, Newspace.im and small launcher companies' websites and user guides.
Evolution of the *cost per kg* of Small Launch Vehicles over time, for both existing and planned concepts:
The physics of launchers is governed by the Rocket Equation:
$$\Delta V = v_e \log(\frac{m_0}{m_f})$$
<math> \Delta V </math> is the change of velocity available to the missile
<math> m_0 </math> is the initial wet mass
<math> m_f </math> is the (final) dry mass
<math> v_e </math> is the effective exhaust velocity
Alignment of Strategic Drivers
We see the competitiveness of the SLV market to be soon dictated by launchers' scheduling flexibility and launch frequency, but with cost per kilogram still as a significant figure of merit. With this in mind, our SLV company aims to allow, first, the highest scheduling flexibility (shortest time between customer contact to launch) and, second, as affordable as possible in these conditions.
| Number | Strategic Objective | Alignment and Target(s) |
|---|---|---|
| 1 | A scheduling flexibility that allows a customer to launch in the shortest time frame after the 1st contact | The current average is ~2 years. A competitive average would be a required time of ~1.5 years. A dominant average would be 6 months. We aim to first reach the scheduling flexibility of 6 months, eventually aiming to achieve a required planning time of only 4 weeks. |
| 2 | A launch frequency that allows us to launch as many payloads from as many customers as possible | The current average is ~2-3 launches per year . A competitive average would be ~6 launches per year. A dominant average would be 10 launches per year. We aim to reach the launch frequency of 10 launches per year. |
| 3 | A total cost that is affordable by even small, academic/organizational endeavors with modest budgets | We aim to be able to perform any launch with a cost per kilogram under $16,000 and a total cost under $11,500,000 (400kg payload at $16,000/kg and $5,000,000 of fixed costs |
FOMs such as reliability and mass to LEO are not included for a reasons. Reliability is of course a significant consideration, but not necessarily a strategic driver that is pursued per se. Of course, with any operations such as rocket launches that have considerable overhead costs and risks of catastrophic failure (entire system loss) it is important to reduce costs risks however possible. However, maximizing reliability and mass to LEO always helps but is not a primary driver of being successful in extracting value from the small launch market.
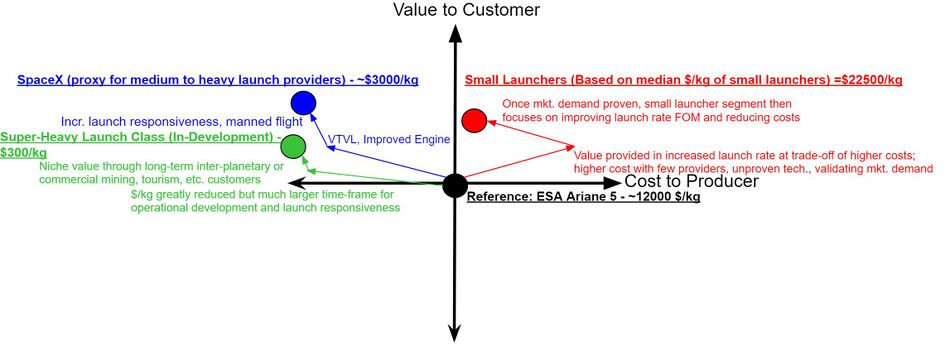
Positioning of Company versus Competition
The first graph depicts the positioning of the small launcher market with respect to the medium, heavy, and super-heavy launch markets. Larger launchers, such as SpaceX's Falcon 9 rocket, are able to provider cheaper costs per kilogram with high reliability which explains their positioning as cheaper producers and improved value. Small launchers on the other hand cost more per kilogram than the other launchers but the launch frequency as well as the responsiveness are improved.
The micro- and nano- launch markets are much smaller and unproven so therefore not shown. However, the value of those two markets would be more niche in providing smaller, more specified payloads and thus more expensive with respect to $/kg albeit it at the tradeoff of likely higher launch frequencies and lower overall costs.
The above graph depicts the positioning of a standard small launch company (based upon the average small launcher cost per kilogram (average frequency of launch is difficult to assume due to low available data but would be a better reference and indicator of value) based on the suggested FOM introduced by small launchers. "Value" in this sense is a combination of various FOMs such as launch frequency and launch costs ($/kg). It is weighted heavily toward launch frequency as this is the primary FOM for small launchers, although launch cost is still significant. As seen, various small launch vehicle providers are attempting to bring value through respectively unique means. Some are attempting to introduce reusable stages similar to SpaceX's Falcon 9 to small launchers, others are attempting to narrow in on the niche of cubesats, some are attempting to focus on seaborne launch which reduces the costs of aborting launch and clearing an area/waiting for weather, and so on. Although some competitors are increasing costs in order to accommodate more niche markets while others are pursuing more advanced, riskier technologies to reduce overall costs, all are attempting to differentiate their service from other competitors in order to extract value from their own niche and remain profitable in their own competitive space.
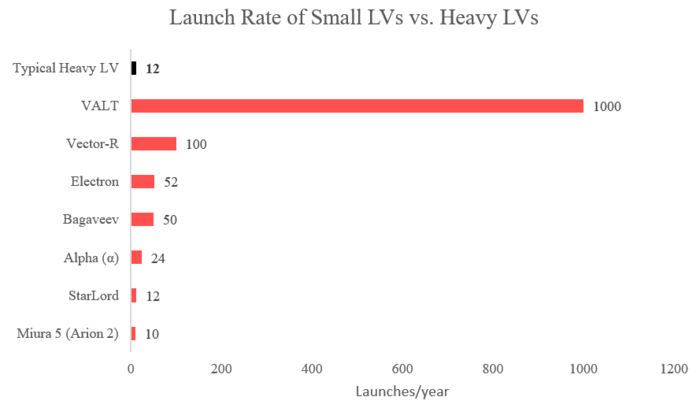
Small Launcher Cost Per Kilogram and Launch Frequency Data Table
| Launcher | Launches Per Year | Cost Per Kilogram ($/kg) |
|---|---|---|
| Vector-R | 100 | 25000 |
| Electron | 52 | 39300 |
| Bagaveev | 50 | 25000 |
| Alpha | 24 | 15000 |
| StarLord | 12 | 20000 |
| Miura 5 (Arion 2) | 10 | 32500 |
| Pythom | 2 | 12000 |
| OS-M | 5 | 16000 |
| Pegasus | 6 | 180586 |
| Kuaizhou-1A | 5 | 20000 |
| JAXA SS-520 | 0.5 | 31429 |
The pareto front above is not based on time but rather cost per kilogram and launches per year (innate with as launch frequency/scheduling flexibility). As seen, the Pythom, Alpha, and Vector-R launchers are pareto optimal designs whereas the other launchers are dominated alternatives. Of note, however, is that many, many launchers (150+, 50 of which are pictured below) did not have data publicly available on both Figures of Merit and therefore were omitted from the data set. Furthermore, some launchers such as Pythom, are still in the developmental stages are projecting these launch costs and frequencies despite having never operationally proven these figures. Other launchers, some as the SS-520, Pegasus, and Kuaizhou-1A have been operationally proven with several successful launches. Currently, small launch companies are being reached out to manually in order to inquire about projections and more data is being continually added to the set as they respond. The current pareto optimal alternatives are expected to shift as more are added and the model can be improved by differentiating between pareto optimal launchers that are operational and those that are not yet launched. Finally, at $22,500, the reference company above would be pareto dominated by all other small launchers currently on the market. This means that the reference company should focus on both decreasing launch costs and increasing launch frequency.
Our database of competing SLV providers:
Technical Model: Morphological Matrix and Tradespace
Morphological Matrix:
The *Scheduling Flexibility* of SLVs (Small Launch Vehicles) is by far the main differentiator of this market segment. Building such a model for responsiveness (which is based on organizational principles rather than physical principles) is complex and very little data is available at this granularity. For these reasons we decomposed this figure of merits into a sum of components on which we have actual data, or can do an educated guess with limited uncertainty in terms of factors at play and relative magnitudes. We used the SLV databases already mentioned in previous sections, manufacturers' websites, and for data only available for heavy launch vehicles, we scaled them.
For simplicity, we decide to use ***lead time*** as a proxy for Scheduling Flexibility: it is defined as the average duration between the order from the customer and the actual launch provided by the SLV services company. It assumes that a contract already exists between the two sides, but the chronometer starts when the actual orbit parameters, launch date and other specifications are communicated. Delays are very frequent, so we decompose responsiveness in the following way:
Lead time (days): $$R = \sum D_{\text{Launch Prep. Phases}} + \sum P_{\text{Delay Likelihood}} \cdot D_{\text{Delay}}$$ $$R = D_{1} + D_{2} + D_{3} + D_{4} + D_{5} + D_{6} + P_L D_{L-Delay} + P_S D_{S-Delay}$$
With (in Days):
S/C mating onto adapter: $$D_{1} = 3 \frac{m}{2000} $$ S/C mating into launcher: $$D_{2} = 5 \frac{m}{2000}$$ Fairing installation $$D_{3} = 1 $$ Launchpad preparation: $$D_{4} = 7 + 3 N + 2 S$$ S/C-Launcher Mission definition (trajectory, perf...): $$D_{5} = 15 - \frac{N}{2} - \frac{3}{2} S$$ Fuel system of main stage: $D_{6} = 2$ for SRMs (solid rocket motors) ; $D_{6} = 6$ for liquid rocket engines
Delays:
- Launcher
Likelihood: $$P_L= 0.3*\frac{m}{2000} + P_1 + P_2 $$ with <math> P_1 = 0.05 </math> if reused launcher (0 otherwise) and $P_2 = 0.1$ for liquid rocket engines (0 otherwise). Delay: $$D_{L-Delay} = 10$$
- Satellite/Spacecraft S/C
Likelihood: $$P_S= 0.05*\frac{m}{2000} $$ Delay: $$D_{L-Delay} = 20$$
Parameters:
m payload mass capacity (mass of the spacecraft, S/C) 0<m<=2000kg
N number of launchpad sites available (hypothesis: optimized spreading among the conventional inclination values used for LEO missions). 0<N<=8
S number of rocket stages 0<S<=3
“𝛿0” Disposable vs reused launcher main stage
“𝛿1” Propellant type for main stage (solid vs liquid)
Comments: Phases that can be conducted in parallel of other “constraining” tasks (i.e. on the critical path of the supply chain) have been included in phases done in series. N is a variable that describes the ability of the company to launch from different sites at different inclinations, whether it is a fixed launchpad or a movable one (the more launchpad sites available, the easier the mission definition phase, the shorter the wait for an available site, but the less ready to go)
As expected, in order to improve the responsiveness (reduce the lead time) of a SLV, it should have: - a lower mass capacity - a lower number of launchpads (but it will restrain of course the type of missions feasible) - a lower number of stages (2, or sometimes 1, instead of 3 for heavy launch vehicles, but it will restrain of course the type of orbit insertions feasible) - Solid propulsion instead of liquid propulsion (which has other inconvenients, such as no restartability) - Disposable main stage (used launchers have generally more unexpected failure, but will save a lot of money on the long term).
Another important FoM for any rocket is its ***Delta V***, which will enable it to reach specific orbits. In the case of SLVs, some rockets will be able to reach 300-400km LEO orbits, while others reach up to more than 1000km LEO orbits. SLVs are usually unable to reach MEO or GEO orbits (Medium Earth Orbit or Geostationary orbit) with they typical payloads.
Rocket equation (neglecting drag and gravity losses): $$\Delta V = \text{Isp} \cdot g_0 \log (R)$$
Parameters:
- Isp the specific impulse of the SLV, which is a measure of how effectively the SLV uses its propellant
- R = M0/Mf is the mass ratio of our rocket, M0 being the wet mass (mass including propellant) and Mf the dry mass (final mass, with all propellant consumed)
$$ \frac{\partial \Delta V}{\partial \text{Isp}} = g_0 \log (R)$$
$$ \frac{\partial \Delta V}{\partial R} = \frac{\text{Isp} \cdot g_0 }{R}$$
$$ \frac{Isp_0}{\Delta V_0} g_0 \log (R_0) = 0.99$$
$$ \frac{R_0}{\Delta V_0} \frac{\text{Isp_0} \cdot g_0 }{R_0} = 0.43 $$
Financial Model
This financial model is performed from the perspective of our launch vehicle services company (model details and hypotheses are stated below):
The breakdown of the expected financial performance brought by the 1SLV roadmap is as follows:
- Annual profit and cumulated profit are derived from sales revenue and costs:
- Revenues
- Revenue sources are contracts with satellite operators to launch their payloads to orbit. Thanks to the responsiveness of our launch vehicle, we assume in our model that the whole payment for a launch is processed within a timeframe of one year (from initial deposits to final payment close to launch date).
- Revenue directly depend on the number of launches performed every year. It is modeled as a slow ramp-up phase (prototype, with lower revenue to account for the high risk of failure), then a phase of acceleration, then convergence to the size of the market segment addressed by our company. Prices have been taken from the analyses conducted above, from our launchers database, and when necessary from the Rocket Lab electron rocket.
- Revenues
- Costs
- Manufacturing cost (variable): modeled in steady range as 80% of the price of the launcher (for the customer - data from electron), with an initial phase of high manufacturing cost to account for growing economies of scale. They start in 2021 with the manufacturing of the first launchers.
- Nonrecurring costs (fixed): investments to develop this new small launch vehicle leveraging the technologies explained above. We assume that the product development process (PDP) was started earlier in 2016, and investments were done over a 6-year period. 2021 is a year of transition where there are still final PDP investments along with variable costs for the manufacturing of the first launchers.
- Costs
This financial model is of course a projection, that should be accompanied by an uncertainty level (see error bars on the graph above). We built a simple error model which accounts for uncertainty in development costs, sales and manufacturing costs. The uncertainty on sales is the main driver of the overall uncertainty level, followed by uncertainty in PDP-related investments, and finally manufacturing costs.
Main insights from the financial analysis:
- The average financial scenario is profitable in 2032, which is long but reasonable for this very specific market of launch vehicles with huge initial investments. It is actually close in timeframe to what was announced by Vector Lab. In the worst case scenario considered, profits only exceed upfront investments in the year 2035 (lower boundary of the last cumulative profit estimate).
- Breakeven happens in 2022, second year after starting manufacturing the rockets
Hence the financial projections (also shown on the graph above):
List of R&T Projects and Prototypes
Summary
The choice of R&D projects to undertake is driven by two different sets of priorities. First, our strategic drivers of increased scheduling flexibility and decreased total cost. Second, those that improve secondary FoMs: launch frequency (launches/month), reliability (% successful launches, payload mass capacity (kg to 500km LEO), and reusability (# of reuse cycles).
R&D Projects
| R&D Project | Technology Area | Description | Pros | Cons | Invest? Y/N |
|---|---|---|---|---|---|
| Additive Manufacturing | Materials | 3D-Printing of rocket components out of metal alloys |
|
|
Y |
| Modular Launchpad | Vehicle Integration | Allows easier integration of launch vehicles into launchpads |
|
|
Y |
| Case Materials | Rigging and Load Integration | Improvement of materials and composites and attachments used in the vessel of the rocket to allow easier integration of payloads and lower mass vehicles as well as more quick, reliable, and/or resilient fabrication |
|
|
Y(/N) |
| Rocket-Based Combined-Cycle | Propulsion System | These projects integrate ramjet and/or scramjet technology with rocket launches in order to improve acceleration to orbit. |
|
|
N |
| Models and Approaches for Remaining Useful Life Prognostics | Prognostic | Allows component level measurement and estimation/prediction of time to failure so that forecasting and mitigation of failures may occur within an acceptable confidence level (90-99%) |
|
|
N |
| Air Launch and Drop System | Plane Launch | Uses fighter or airliner planes to reach a higher altitude before launching the attached payload |
|
|
N |
A comparison of the R&D projects that are projected to alter the FOMs significantly, the scramjet and air launch projects, is below. Compared to traditional rockets, they appear to come vastly closer to the utopia point. However, there are no legitimate, successful tests of either of these. Although the prospects are promising, the TRL is simply too low and the cost of investment to increase this to operational capabilities is too high. However, as the TRL increases through research currently being performed by other agencies, primarily the Department of Defense, we recognize these technologies as potentially threatening to the small launch vehicle market space we currently operate in.
Other R&D projects - prognostics model, case materials, and modular launchpad - have higher TRLs but have less significant impacts on the key FOMs, most importantly the scheduling flexibility and total cost. The prognostic model is being developed by other companies that also incorporate reusable boosters as this technology would be particularly beneficial to assess the reused boosters. Since there are no plans to explore the reusable booster technology space, we do not see this an optimal choice to invest in currently.
Investing in case materials and a modular launch pad are the most lucrative as these contribute to the FOM most relevant to our business model of appealing to consumers that would prefer to be able to launch on demand rather than wait months/years for cheaper ridesharing options. A modular launchpad and revised case materials allow clients to more easily integrate payloads into our launch vehicles, thereby resulting in increased scheduling flexibility (shorter time between initial talks to launch). As our primary FOM and a central aspect to our business model, the benefits yielded from both of these R&D projects that are also relatively inexpensive to design and develop, warrant the further examination below.
The values are gathered from current cost estimations for related technologies combined into a nominal projected value. For example, the cost estimation for a modular launchpad was based on the current TRL of modular launchpads based upon NASA publications, the current cost of a launchpad, and the current cost of similar R&D projects for materials and structures research. It should be noted that we assume diminishing returns when all three R&D projects are incorporated simultaneously. This is due to some overlap such in the improvements associated with each. For example, case materials may overlap with the materials science involved in additive manufacturing. Our company's technology baseline is a rounded down average of the three pareto dominant alternatives analyzed above: Pythom, Firefly (Alpha), and Vector-R. This makes us near competitive, but just under pareto dominant without any R&D investments. In order to reach pareto dominance, and by extension a competitive market position, we chose to invest in the aforementioned R&D projects with higher TRLs that can become operational in a shorter amount of time. This, in turn, allows us to recoup our investment more quickly. The cost estimations for these projects are based on data available from other sectors attempting to incorporate such projects into their own products (e.g. additive manufacturing in robotics, planes, and construction yielded baseline data for us to estimate the cost and value added for the small launch market). Our pre-research hypothesis that the value-added from these projects is low seems to be correct as the pareto frontier shifts only slightly. However, this shift is enough to exclude Firefly (Alpha) from being a pareto dominant competitor and makes our costs competitive with the others launch providers. It can also be observed that our R&D costs associated with becoming a pareto dominant competitor can be recouped in just one year of launched, assuming we are able to complete all 70 launches expected.
Key Publications and Patents
Number of publications per year about small launch vehicles (and equivalent denominations):
Most families of launch vehicles started as small launcher prototypes, as early as the 50s/60s, hence the few papers from that period. However, small launchers really became an attractive design in the 90s with the start of the miniaturization of satellites. There is a growing number of publications every year on the topic. If we conduct an analysis of author affiliations, we note that JPL (CA) is by far the most active on this topic, followed by the China Academy of Launch Vehicle Technology, and NASA. The countries of origin are the following:
We decided to focus on two papers about small launch vehicle technologies, highly cited and giving a good overview of current research areas. The first focuses on the use of small launchers for cubesat constellations’ deployment, the second one focuses on vibration isolation to protect payloads during launch from SLVs.
“Utilizing Small Launch Vehicles for Multiple Small Payload Missions” (2017)
Sending several cubesat payloads with different orbital parameters in a single launch is a challenge today because these often don’t have on-board propulsion systems and because small rockets don’t have 3rd/4th stages to precisely insert satellites one at a time. This paper presents a concept for a low-cost/low-mass “propulsive rideshare adapter” called SL-OMV that is able to remain on orbit for longer durations to distribute cubesats up to 6U to their respective LEO orbits. Both authors are employees of Moog, Inc (Aerospace and Defense company). Their solution may be particularly helpful for the deployment of cubesat constellations.
SL-OMV from below (left) and integrated under the fairings (right). SL-OMV Capacities.
“Multi-axis whole-spacecraft vibration isolation for small launch vehicles” (2001)
Vibration isolation systems are critical for launch vehicles to protect their sensitive payloads from vibrations resulting from the launch. Because of the mass and volume constraints on small launch vehicles, conventional isolation systems cannot be used. This is clearly a threat to the attraction of small launcher vehicles. This paper presents two passive vibration isolation systems to reduce dynamic launch loads. The first one protects against axial loads and has been used in three launches to date. The second one is a multi-axis device that performs the same functions and has been tested twice for space missions. They are both relatively simple to manufacture, passive, small, lightweight and reliable.
Additional attenuation provided by the second isolation device (accelerations/frequencies).
Patent analysis
We have found patents from small launch vehicle startups, aerospace companies and agencies about propulsion technologies, avionics, control software, launch pads and manufacturing methods. Most patents were filed during the past two decades. The first patent shows a way for SLV manufacturers to cut costs associated with high-quality avionics systems onboard launchers. The second one provides a mechanism to save mass and volume in the satellite deployment system for SLVs.
“Affordable vehicle avionics system”, 2020, NASA
Developed at NASA Ames research center, the AVA (Affordable Vehicle Avionics) is a low-cost, self contained GNC (Guidance, Navigation and Control) system made for nano- and micro-launchers. This system is qualified to survive the launch environment and leverages already existing commercial sensors and processors, to make it cost-effective (10% of the current cost of such systems). Its size is 100 mm x 120 mm x 69 mm with a mass of 0.84kg, it includes a tactical-grade MEMS inertial measurement unit that leverages a GPS receiver, inertial sensors and a magnetometer to reach precision levels suited for space navigation.
CAD model and pictures of the AVA (NASA website)
“Triggered satellite deployment mechanism”, 2016, Vector Launch Inc.
This patent presents an improved design for payload deployment systems. Weight and size are even more critical for small launch vehicles than for traditional launch vehicles, so this patent minimizes the size of the deployment mechanism. More precisely, it avoids having to enclose the satellite in a box structure. It instead uses pairs of “opposing hooks that slide over a set of small chassi rails” that stick out and are compliant with cubesat standards.
Satellite deployment mechanism