Difference between revisions of "Mobile Nuclear Fission Reactors"
| Line 185: | Line 185: | ||
'''Theoretical limit''' | '''Theoretical limit''' | ||
The theoretical limit for efficiency is 0<<1. The lower bound limit of the scale where =0 suggests that none of the thermal power generated by the reactor is converted to electrical power. The upper bound limit of the scale where =1 suggests that all the thermal power generated by the reactor is converted to electrical power. Due to the conservation of energy, it is theoretically not possible for | |||
We note that there is a limitation to the linear model shown in Figure 1 because it indicates that reactor efficiency can be greater than the theoretical upper bound of 1 in the far future. Thus, the linear model may only be valid to project reactor efficiency in the short term (e.g. 5-10 years ahead). | The theoretical limit for reactor efficiency is '''0 < efficiency < 1'''. The lower bound limit of the scale where effiency = 0 suggests that none of the thermal power generated by the reactor is converted to electrical power. The upper bound limit of the scale where efficiency = 1 suggests that all the thermal power generated by the reactor is converted to electrical power. Due to the conservation of energy, it is theoretically not possible for effiency > 1 because this would indicate that energy is being produced in the process of converting a fixed amount of thermal energy to electrical energy. The efficiency also cannot be less than 0 because this indicates that energy is being consumed in the process of converting a fixed amount of thermal energy to electrical energy. We note that there is a limitation to the linear model shown in Figure 1 because it indicates that reactor efficiency can be greater than the theoretical upper bound of 1 in the far future. Thus, the linear model may only be valid to project reactor efficiency in the short term (e.g. 5-10 years ahead). | ||
Revision as of 03:24, 11 October 2023
Roadmap Overview
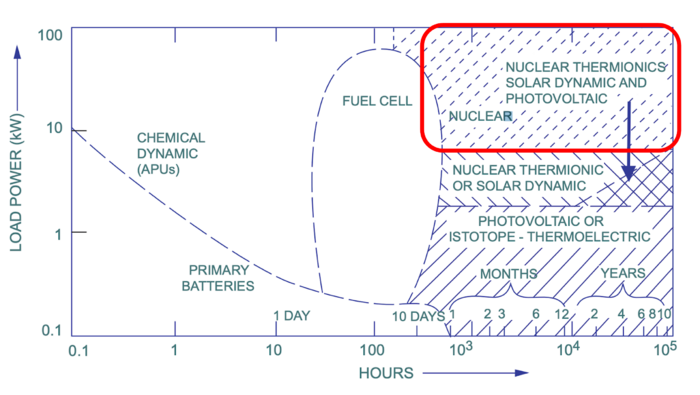
As humans venture further into space beyond low Earth orbit to establish settlements, there emerges a need for reliable power generation technologies for human settlements on other planets. While photovoltaics, fuel cells, batteries, and radioisotope thermoelectric generators (RTGs) are considered possible power sources, each have limitations within the scope of long duration missions that require higher loads and reliability to support human settlements. For example, solar arrays are highly dependent on the sun, meaning that it cannot generate power during night times. Batteries generally provide low power and have a short lifespan, making them unsuitable power sources for human settlements. As shown in the diagram below, the need for higher load powers and longer duration missions points to nuclear energy as a source of power for human settlements on other planets. The Mobile Nuclear Fission Reactor (MNFR) is a technology that can provide nuclear energy and will be the focus of this technology roadmap. It is important that we describe the nuclear fission reactor technology being roadmapped as being “Mobile” because it must be able to move freely and easily so that it can be transported from Earth to the planet via a launch vehicle.
Image taken from: https://ocw.mit.edu/courses/16-851-satellite-engineering-fall-2003/2c8a1a136db0e366ae8d1a1e2995a505_l3_scpowersys_dm_done2.pdf
At high-level, nuclear fission reactors generate power through the nuclear fission chain reaction of fuel that is stored within the reactor core. The fuel contains fissile isotopes of elements such as uranium-235 and plutonium-239. The reactor core generates heat as a result of the chain reaction, and the heat is converted into kinetic energy and ultimately electrical energy that is distributed and used. Using nuclear fission reactors in extraterrestrial environments imposes constraints on the system which are inherently different from Earth-based reactor systems. Some examples of these constraints include the reactor size/mass (due to launch costs), safety, decommissioning, and cost of operations. This roadmap will allow us to project future nuclear fission reactor capabilities that we may potentially harness to generate power for future human settlements in space.
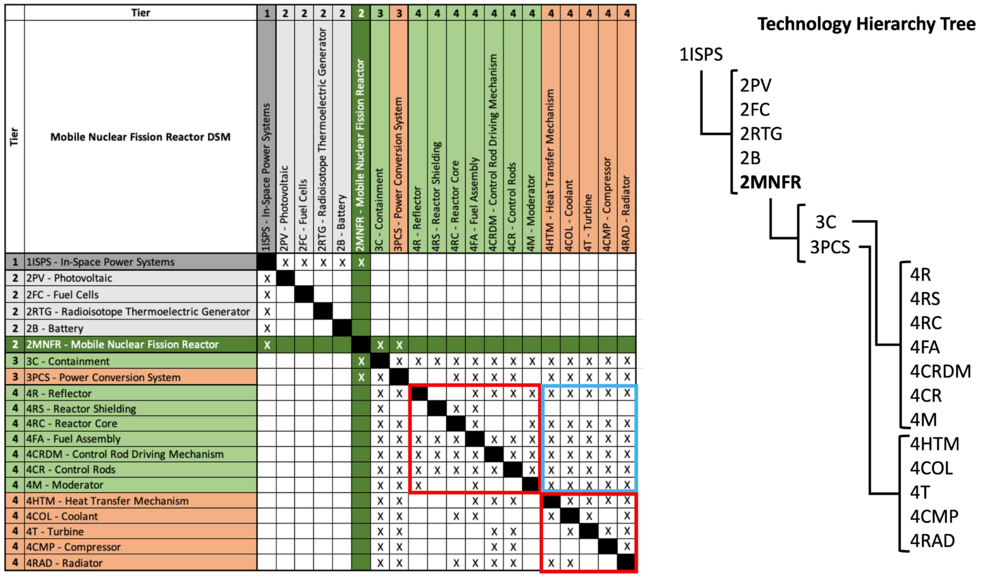
Design Structure Matrix (DSM) Allocation
The Mobile Nuclear Fission Reactor is identified to be a tier two technology because it is part of a wider initiative to develop power technologies (such as fuel cells, photovoltaics etc.) that can support in-space power systems for human habitats on other planetary surfaces. The Mobile Nuclear Fission Reactor (2-MNFR) is highlighted in dark green in the DSM below on the left. The MNFR is then broken down into two tier three systems which includes the containment and power conversion system. The containment and power conversion systems are then further broken down into tier four subsystems as summarized in the technology hierarchy tree below on the right.
The DSM highlights key dependencies between systems that make up the MNFR. Two notable observations could be made:
1.There is high interdependency between components that comprise the containment system and components that comprise the power conversion system. This is highlighted by the red boxes shown in the DSM. This outcome is expected because the tier four components in a reactor are designed to have a coupled relationship for the reactor to function
2. There is a high level of cross-system dependency between the containment system and power conversion system where the power conversion system receives “inputs” from the containment system. This outcome is expected because in a reactor, the power conversion system is downstream of the containment system (i.e.: the containment system produces thermal energy, and the power conversion system turns that into electrical energy). This cross-system interdependency is highlighted by the blue box shown in the DSM.
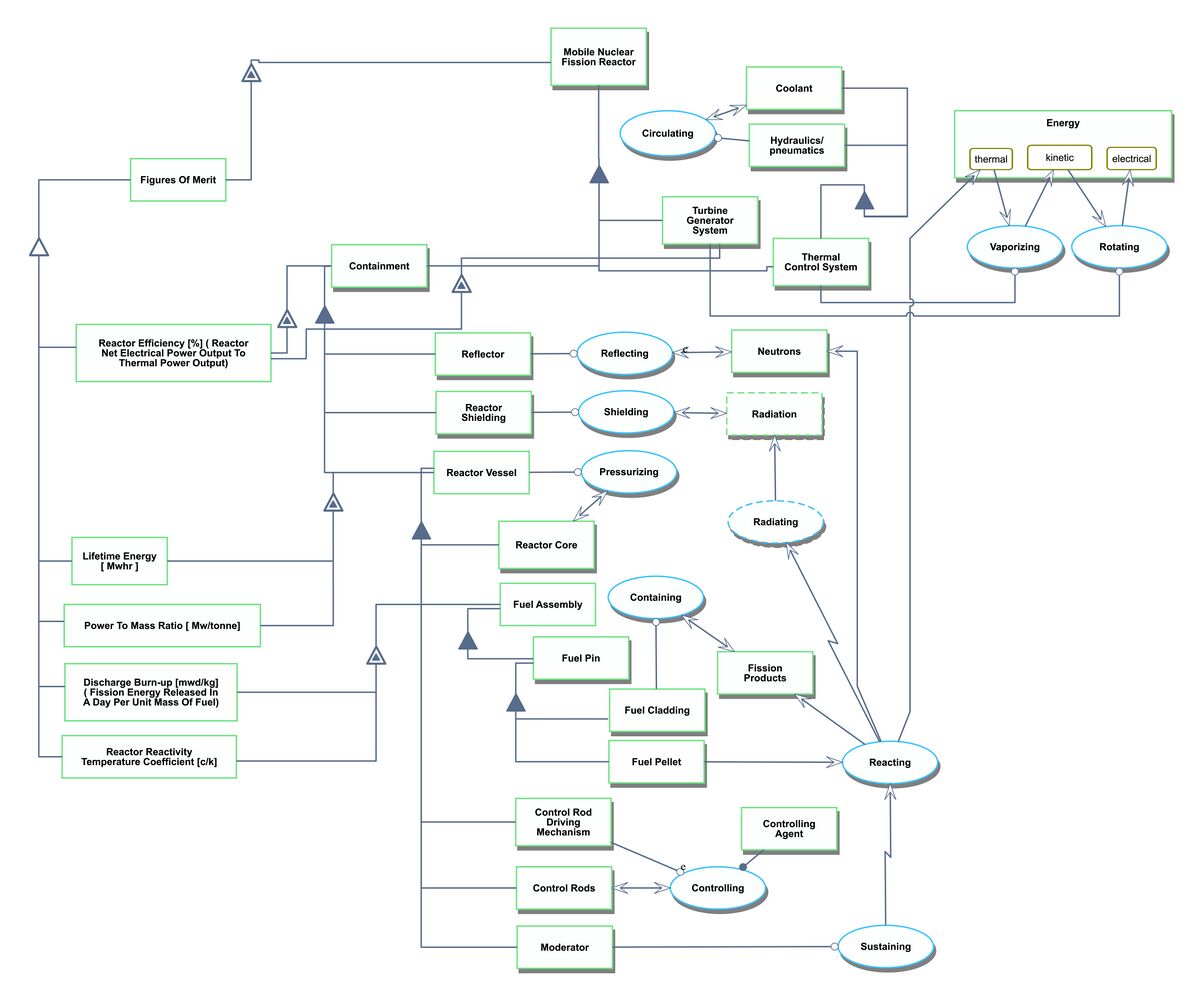
Roadmap Model using OPM
Shown below is an OPD of the Mobile Nuclear Fission Reactor system that was generated by OPCloud. The OPD captures the figures of merit, processes, and components involved with a MNFR. The key relationship to note from the OPD is that the containment system generates thermal energy and the power conversion system (which refers to the turbine generator system and thermal control system combined) converts the thermal energy to kinetic energy and then to electrical energy.
Figures of Merit (FOM)
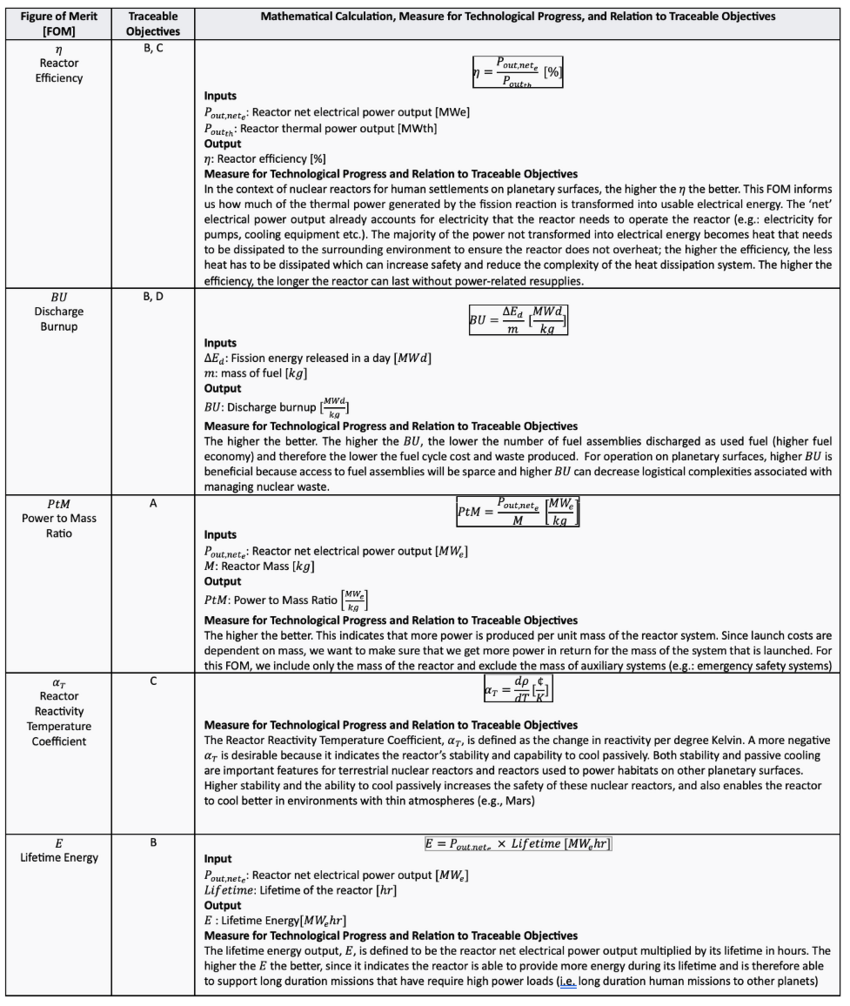
The following FOMs were selected to track the technological progress of MNFRs over time.
| Figure of Merit | Units | Description |
|---|---|---|
| Reactor Efficiency | [%] | Proportion of electrical power generated from thermal power generated |
| Discharge Burnup | [MWd/kg] | Fission energy released in a day per unit mass of the fuel |
| Power to Mass Ratio | Unitless | Electrical power generated per unit mass of the system |
| Reactor Reactivity Temperature Coefficient | [c/K] | Change in reactivity per degree Kelvin |
It is important that the figures of merit selected are traceable to high-level stakeholder objectives which are shown below for different phases of a mission involving MNFRs to provide power for human habitats on other planetary surfaces:
Launch
[A] The system should target to have as low of a mass it can for the amount of power it can generate because launch costs are dependent on mass.
Operation
[B] The system should target to be self-sustaining for long periods of time because maintenance/resupply capabilities are more costly and complex the farther the farther away the proposed habitat is from Earth.
[C] The system should be operated safely
End of Life
[D] The system should aim to have low waste production since nuclear waste is hard to manage.
The table below describes each FOM mathematically and indicates which objective they are traceable to. It also discusses whether higher/lower values of each FOM indicate technological progress and how each FOM is related to the objective it traces.
Tracking FOMs over Time [dFOM/dt]
Background information and data was collected for the reactor efficiency FOM, which is described mathematically in the table above. Relevant data was collected for 11 space reactor power systems between the years 1964 to 2018 as shown below.
Figure 1. Plot showing the reactor efficiency over time for eleven space reactor power systems.
When a linear trend line is fitted to the data points, the equation yielded is:
y = 0.0049x - 9.5428 with an R^2 value of 0.7061
The gradient of the trendline (0.0049) indicates that the linear rate of improvement is 0.49% per year. The R2 value of 0.7061 generally indicates a good fit of the linear model to the data.
Theoretical limit
The theoretical limit for reactor efficiency is 0 < efficiency < 1. The lower bound limit of the scale where effiency = 0 suggests that none of the thermal power generated by the reactor is converted to electrical power. The upper bound limit of the scale where efficiency = 1 suggests that all the thermal power generated by the reactor is converted to electrical power. Due to the conservation of energy, it is theoretically not possible for effiency > 1 because this would indicate that energy is being produced in the process of converting a fixed amount of thermal energy to electrical energy. The efficiency also cannot be less than 0 because this indicates that energy is being consumed in the process of converting a fixed amount of thermal energy to electrical energy. We note that there is a limitation to the linear model shown in Figure 1 because it indicates that reactor efficiency can be greater than the theoretical upper bound of 1 in the far future. Thus, the linear model may only be valid to project reactor efficiency in the short term (e.g. 5-10 years ahead).