Satellite Autonomous Systems

Satellite Autonomous Systems for Rendezvous and Proximity Operations

Roadmap Overview

There is a need for satellites designed to perform a wide range of tasks in space without constant human intervention, including navigation, data collection, decision-making, rendezvous, proximity operations, docking, debris disposal, and servicing. We present a strategic plan supported by a technological pathway for the development and deployment of satellites capable of operating autonomously in space.

Autonomous satellites will be equipped with advanced onboard systems that allow them to navigate and adjust their orbits, avoiding collisions with other space objects or debris, enhancing the efficacy of space missions. Advanced onboard processing is needed for real time decision making that allows for responses to dynamic situations and course correction, particularly in rendezvous and proximity ops. The ability communicate and make decisions autonomously with information shared between satellites is valuable for many mission types including In-Space Servicing, Assembly, and Manufacturing (ISAM), Active Debris Removal (ADR), and constellations of satellites working together in formation flight.

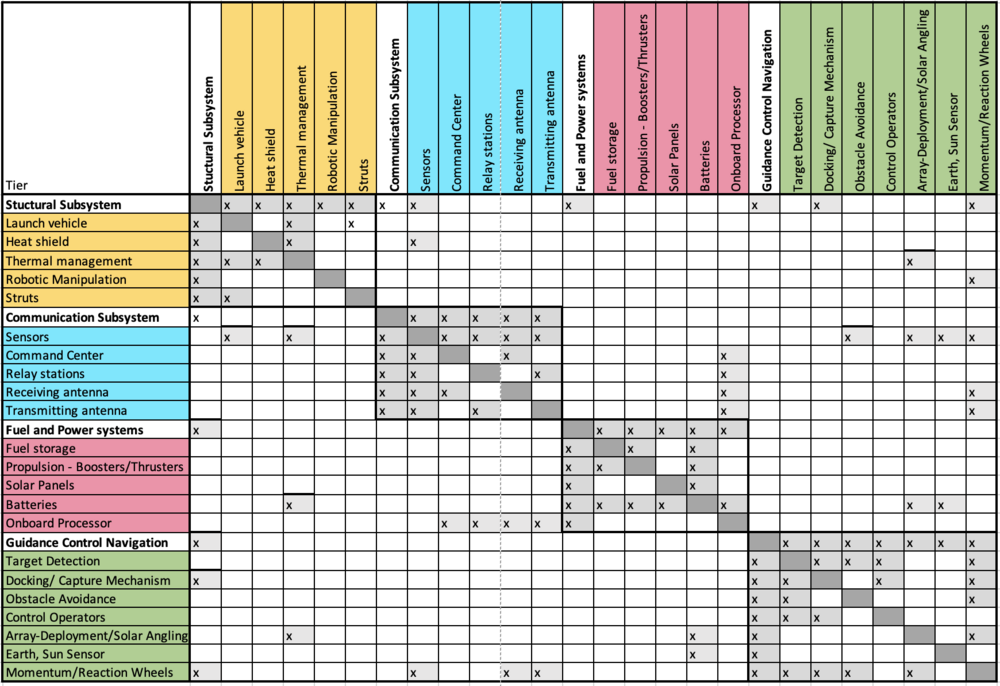
DSM Allocation

OPM

Figures of Merit (FOM)

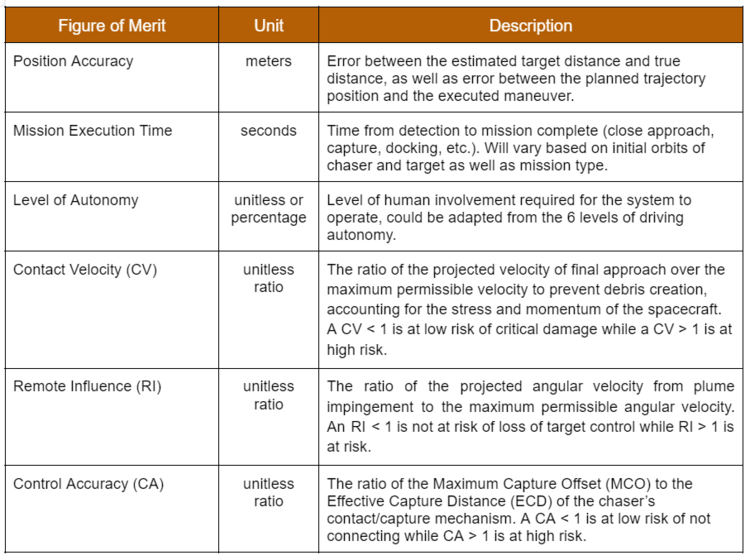
Autonomous systems for Rendezvous and Proximity Operations (RPO) can be utilized for a number of spacecraft missions, including inspection, capture, docking, servicing, formation flight, etc. Given this variety, it is difficult to standardize FOMs among all mission types. Some proposed FOMs are identified in Table 2.

Proposed Safety-Related FOMS A survey of missions which included elements of RPO published in 2018 and performed by the University of Southern California’s (USC) Space Engineering Research Center (SERC) in association with the Consortium for Execution of Rendezvous and Servicing Operations (CONFERS) sought to identify potential attributes for comparison in order to define safe standards for RPO. The survey found that there were only a few comparable attributes with no discernable pattern other than proposed ‘safety nets’ around targets during approach, which could vary widely among mission types. However, there was agreement that these systems should perform accurately while applying the principle of ‘do not harm’ to the space environment by preventing debris creating collisions. The SERC therefore proposed three metrics to address safety within the final approach of RPO: Contact Velocity, Remote Influence, and Control Accuracy. These metrics are unitless ratios and therefore independent of scale and are assumed to be applied to two spacecraft (a chaser and target); so they are not necessarily applicable to RPO with pieces of debris.

Contact Velocity is based on the practice of maintaining a relative velocity to the target such that if no braking maneuver is executed, the collision of the spacecraft will not result in separable debris from either. It is defined as the ratio of the projected velocity of final approach over the maximum permissible velocity to prevent debris creation, accounting for the stress and momentum of the spacecraft. A CV < 1 is at low risk of critical damage while a CV > 1 is at high risk.

Remote Influence is based on the practice of maintaining a distance between the spacecraft and orientation such that forces from the chaser spacecraft (like thruster plume) don’t exert unintended torque on the target. It is defined as the ratio of the projected angular velocity from plume impingement to the maximum permissible angular velocity. An RI < 1 is not at risk of loss of target control while RI > 1 is at risk.

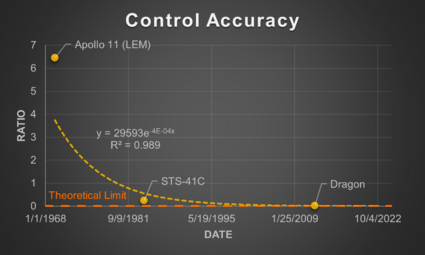
Control Accuracy is mainly related to mission types where connection between spacecraft is intended and is based on the practice of maintaining control of the chaser such that the worst-case offset error does not exceed the Effective Capture Distance (ECD) of the chaser’s contact/capture mechanism. It is defined as the ratio of the Maximum Capture Offset (MCO) to the ECD. A CA < 1 is at low risk of not connecting while CA > 1 is at high risk.

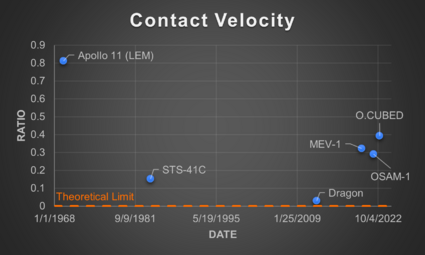
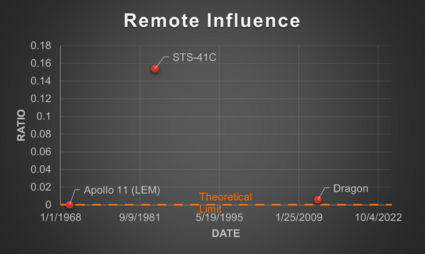
Using the results from the survey, the SERC calculated these metrics for three example RPO missions shown in Table 2. Contact Velocity was calculated for three additional missions in a subsequent paper (Note: RESTORE-L is now the OSAM-1 mission).

When graphed, these FOMs exhibit interesting characteristics related to technological development. The theoretical limit for each of them is 0 since this when no potential inadvertent force or contact would occur. Looking further at the Control Accuracy FOM, this does seem to exhibit a potential part of an inverted S-Curve, though there are too few data points to confirm. Based on this, however, we may estimate that the technology is currently in the slowing to stagnation phase of the technology development. We can also perhaps see a partial of an inverted S-Curve when looking at the Contact Velocity FOM, though it is thrown off by the newer missions. This could be a result though of the different risk tolerances of the mission types (human carrying vs. not) that lead to certain design choices which influence the FOM.

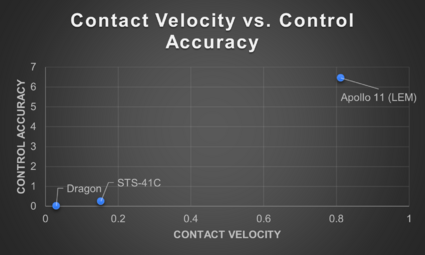
When Contact Velocity vs. Control Accuracy is graphed, one can see a possible Pareto Shift Model, with each newer system shifting lower.

In theory, there is a physical limit to the positional accuracy for autonomous systems using optical sensors, and this limit is mainly determined by the laws of physics, particularly the diffraction limit. The diffraction limit defines the smallest resolvable details or features that an optical or radio system can distinguish. This limit is a fundamental constraint on all optical systems, including space cameras, telescopes, and sensors.

The diffraction limit, which defines the minimum resolvable angle (θ) for a circular aperture, is given by the formula:

To evaluate the theoretical limit to remotely sensed location (which affects our FOM of accuracy), let's assume we have a radar system operating at a wavelength of 1 centimeter (0.01 meters) with an antenna size of 5 meters. If we are observing an object 10,000 kilometers away using a radar system with a specific wavelength and antenna size, we can use this formula to calculate the angular resolution. Using the formula:

This translates to an positional accuracy within .00244rad*10000km= 24.4km